Fracture toughness
In materials science, fracture toughness is the critical stress intensity factor of a sharp crack where propagation of the crack suddenly becomes rapid and unlimited. A component's thickness affects the constraint conditions at the tip of a crack with thin components having plane stress conditions and thick components having plane strain conditions. Plane strain conditions give the lowest fracture toughness value which is a material property. The critical value of stress intensity factor in mode I loading measured under plane strain conditions is known as the plane strain fracture toughness, denoted .[1] When a test fails to meet the thickness and other test requirements that are in place to ensure plane strain conditions, the fracture toughness value produced is given the designation . Fracture toughness is a quantitative way of expressing a material's resistance to crack propagation and standard values for a given material are generally available.
Slow self-sustaining crack propagation known as stress corrosion cracking, can occur in a corrosive environment above the threshold and below . Small increments of crack extension can also occur during fatigue crack growth, which after repeated loading cycles, can gradually grow a crack until final failure occurs by exceeding the fracture toughness.
Material variation
| Material type | Material | KIc (MPa · m1/2) |
|---|---|---|
| Metal | Aluminum | 14–28 |
| Aluminum alloy (7075) | 20-35[2] | |
| Inconel 718 | 73-87[3] | |
| Maraging steel (200 Grade) | 175 | |
| Steel alloy (4340) | 50 | |
| Titanium alloy | 84–107[4] | |
| Ceramic | Aluminum oxide | 3–5 |
| Silicon carbide | 3–5 | |
| Soda-lime glass | 0.7–0.8 | |
| Concrete | 0.2–1.4 | |
| Polymer | Polymethyl methacrylate | 0.7–1.60 |
| Polystyrene | 0.7–1.1 | |
| Composite | Mullite-fibre composite | 1.8–3.3[5] |
| Silica aerogels | 0.0008–0.0048[6] |
Fracture toughness varies by approximately 4 orders of magnitude across materials. Metals hold the highest values of fracture toughness. Cracks cannot easily propagate in tough materials, making metals highly resistant to cracking under stress and gives their stress–strain curve a large zone of plastic flow. Ceramics have a lower fracture toughness but show an exceptional improvement in the stress fracture that is attributed to their 1.5 orders of magnitude strength increase, relative to metals. The fracture toughness of composites, made by combining engineering ceramics with engineering polymers, greatly exceeds the individual fracture toughness of the constituent materials.
Mechanisms
Intrinsic mechanisms
Intrinsic toughening mechanisms are processes which act ahead of the crack tip to increase the material's toughness. These will tend to be related to the structure and bonding of the base material, as well as microstructural features and additives to it. Examples of mechanisms include
- crack deflection by secondary phases,
- crack bifurcation due to fine grain structure
- changes in the crack path due to grain boundaries
Any alteration to the base material which increases its ductility can also be thought of as intrinsic toughening.[7]
Grain boundaries
The presence of grains in a material can also affect its toughness by affecting the way cracks propagate. In front of a crack, a plastic zone can be present as the material yields. Beyond that region, the material remains elastic. The conditions for fracture are the most favorable at the boundary between this plastic and elastic zone, and thus cracks often initiate by the cleavage of a grain at that location.
At low temperatures, where the material can become completely brittle, such as in a body-centered cubic (BCC) metal, the plastic zone shrinks away, and only the elastic zone exists. In this state, the crack will propagate by successive cleavage of the grains. At these low temperatures, the yield strength is high, but the fracture strain and crack tip radius of curvature are low, leading to a low toughness.[8]
At higher temperatures, the yield strength decreases, and leads to the formation of the plastic zone. Cleavage is likely to initiate at the elastic-plastic zone boundary, and then link back to the main crack tip. This is usually a mixture of cleavages of grains, and ductile fracture of grains known as fibrous linkages. The percentage of fibrous linkages increase as temperature increases until the linkup is entirely fibrous linkages. In this state, even though yield strength is lower, the presence of ductile fracture and a higher crack tip radius of curvature results in a higher toughness.[8]
Inclusions
Inclusions in a material such as a second phase particles can act similar to brittle grains that can affect crack propagation. Fracture or decohesion at the inclusion can either be caused by the external applied stress or by the dislocations generated by the requirement of the inclusion to maintain contiguity with the matrix around it. Similar to grains, the fracture is most likely to occur at the plastic-elastic zone boundary. Then the crack can linkup back to the main crack. If the plastic zone is small or the density of the inclusions is small, the fracture is more likely to directly link up with the main crack tip. If the plastic zone is large, or the density of inclusions is high, additional inclusion fractures may occur within the plastic zone, and linkup occurs by progressing from the crack to the closest fracturing inclusion within the zone.[8]
Transformation toughening
Transformation toughening is a phenomenon whereby a material undergoes one or more martensitic (displacive, diffusionless) phase transformations which result in an almost instantaneous change in volume of that material. This transformation is triggered by a change in the stress state of the material, such as an increase in tensile stress, and acts in opposition to the applied stress. Thus when the material is locally put under tension, for example at the tip of a growing crack, it can undergo a phase transformation which increases its volume, lowering the local tensile stress and hindering the crack's progression through the material. This mechanism is exploited to increase the toughness of ceramic materials, most notably in Yttria-stabilized zirconia for applications such as ceramic knives and thermal barrier coatings on jet engine turbine blades.[9]
Extrinsic mechanisms
Extrinsic toughening mechanisms are processes which act behind the crack tip to resist its further opening. Examples include
- fibre/lamella bridging, where these structures hold the two fracture surfaces together after the crack has propagated through the matrix,
- crack wedging from the friction between two rough fracture surfaces, and
- microcracking, where smaller cracks form in the material around the main crack, relieving the stress at the crack tip by effectively increasing the material's compliance.[10]
Test methods
Fracture toughness tests are performed to quantify the resistance of a material to failure by cracking. Such tests result in either a single-valued measure of fracture toughness or in a resistance curve. Resistance curves are plots where fracture toughness parameters (K, J etc.) are plotted against parameters characterizing the propagation of crack. The resistance curve or the single-valued fracture toughness is obtained based on the mechanism and stability of fracture. Fracture toughness is a critical mechanical property for engineering applications. There are several types of test used to measure fracture toughness of materials, which generally utilise a notched specimen in one of various configurations. A widely utilized standardized test method is the Charpy impact test whereby a sample with a V-notch or a U-notch is subjected to impact from behind the notch. Also widely used are crack displacement tests such as three-point beam bending tests with thin cracks preset into test specimens before applying load.
Choice of specimen
The ASTM standard E1820 for the measurement of fracture toughness[11] recommends three coupon types for fracture toughness testing, the single-edge bending coupon [SE(B)], the compact tension coupon [C(T)] and the disk-shaped compact tension coupon [DC(T)]. Each specimen configuration is characterized by three dimensions, namely the crack length (a), the thickness (B) and the width (W). The values of these dimensions are determined by the demand of the particular test that is being performed on the specimen. The vast majority of the tests are carried out on either compact or SENB configuration. For the same characteristic dimensions, compact configuration takes a lesser amount of material compared to SENB.
Material orientation
Orientation of fracture is important because of the inherent non-isotropic nature of most engineering materials. Due to this, there may be planes of weakness within the material, and crack growth along this plane may be easier compared to other direction. Due to this importance ASTM has devised a standardized way of reporting the crack orientation with respect to forging axis.[12] The letters L, T and S are used to denote the longitudinal, transverse and short transverse directions, where the longitudinal direction coincides with forging axis. The orientation is defined with two letters the first one being the direction of principal tensile stress and the second one is the direction of crack propagation. Generally speaking, the lower bound of the toughness of a material is obtained in the orientation where the crack grows in the direction of forging axis.
Pre-cracking
For accurate results, a sharp crack is required before testing. Machined notches and slots do not meet this criterion. The most effective way of introducing a sufficiently sharp crack is by applying cyclic loading to grow a fatigue crack from a slot. Fatigue cracks are initiated at the tip of the slot and allowed to extend until the crack length reaches its desired value.
The cyclic loading is controlled carefully so as to not affect the toughness of the material through strain-hardening. This is done by choosing cyclic loads that produce a far smaller plastic zone compared to plastic zone of the main fracture. For example, according to ASTM E399, the maximum stress intensity Kmax should be no larger than 0.6 during the initial stage and less than 0.8 when crack approaches its final size.[13]
In certain cases grooves are machined into the sides of a fracture toughness specimen so that the thickness of the specimen is reduced to a minimum of 80% of the original thickness along the intended path of crack extensions.[14] The reason is to maintain a straight crack front during R-curve test.
The four main standardized tests are described below with KIc and KR tests valid for linear-elastic fracture mechanics (LEFM) while J and JR tests valid for elastic-plastic fracture mechanics (EPFM)
Determination of plane strain fracture toughness
When a material behaves in a linear elastic way prior to failure, such that the plastic zone is small compared to the specimen dimension, a critical value of Mode-I stress intensity factor can be an appropriate fracture parameter. This method provides a quantitative measure of fracture toughness in terms of the critical plane strain stress intensity factor. The test must be validated once complete to ensure the results are meaningful. The specimen size is fixed, and must be large enough to ensure plane strain conditions at the crack tip.
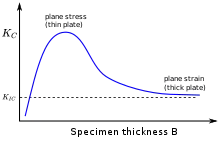
The specimen thickness affects the degree of constraint at the crack tip which in turn affects the fracture toughness value Fracture toughness decreases with increasing specimen size until a plateau is reached. Specimen size requirements in ASTM E 399 are intended to ensure that measurements correspond to the plane strain plateau by ensuring that the specimen fractures under nominally linear elastic conditions. That is, the plastic zone must be small compared to the specimen cross section. Four specimen configurations are permitted by the current version of E 399: the compact, SE(B), arc-shaped, and disk-shaped specimens. Specimens for tests are usually fabricated with the width W equal to twice the thickness B. They are fatigue pre-cracked so that the crack length/width ratio (a /W) lies between 0.45 and 0.55. Thus, the specimen design is such that all of the key dimensions, a, B, and W−a, are approximately equal. This design results in the efficient use of material, since the standard requires that each of these dimensions must be large compared to the plastic zone.
- Plane-strain fracture toughness testing
When performing a fracture toughness test, the most common test specimen configurations are the single edge notch bend (SENB or three-point bend), and the compact tension (CT) specimens. Testing has shown that plane-strain conditions generally prevail when:[15]
where is the minimum necessary thickness, the fracture toughness of the material and is the material yield strength.
The test is performed by loading steadily at a rate such that KI increases from 0.55 to 2.75 (MPa)/s. During the test, the load and the crack mouth opening displacement (CMOD) is recorded and the test is continued till the maximum load is reached. The critical load <PQ is calculated through from the load vs CMOD plot. A provisional toughness KQ is given as
- .
The geometry factor is a dimensionless function of a/W and is given in polynomial form in the E 399 standard. The geometry factor for compact test geometry can be found here.[16] This provisional toughness value is recognized as valid when the following requirements are met:
- and
When a material of unknown fracture toughness is tested, a specimen of full material section thickness is tested or the specimen is sized based on a prediction of the fracture toughness. If the fracture toughness value resulting from the test does not satisfy the requirement of the above equation, the test must be repeated using a thicker specimen. In addition to this thickness calculation, test specifications have several other requirements that must be met (such as the size of the shear lips) before a test can be said to have resulted in a KIC value.
When a test fails to meet the thickness and other plain-strain requirements, the fracture toughness value produced is given the designation Kc. Sometimes, it is not possible to produce a specimen that meets the thickness requirement. For example, when a relatively thin plate with high toughness is being tested, it might not be possible to produce a thicker specimen with plane-strain conditions at the crack tip.
Determination of R-curve, K-R
The specimen showing stable crack growth shows an increasing trend in fracture toughness as the crack length increases (ductile crack extension). This plot of fracture toughness vs crack length is called the resistance (R)-curve. ASTM E561 outlines a procedure for determining toughness vs crack growth curves in materials.[17] This standard does not have a constraint over the minimum thickness of the material and hence can be used for thin sheets however the requirements for LEFM must be fulfilled for the test to be valid. The criteria for LEFM essentially states that in-plane dimension has to be large compared to the plastic zone. There is a misconception about the effect of thickness on the shape of R curve. It is hinted that for the same material thicker section fails by plane strain fracture and shows a single-valued fracture toughness, the thinner section fails by plane stress fracture and shows the rising R-curve. However, the main factor that controls the slope of R curve is the fracture morphology not the thickness. In some material section thickness changes the fracture morphology from ductile tearing to cleavage from thin to thick section, in which case the thickness alone dictates the slope of R-curve. There are cases where even plane strain fracture ensues in rising R-curve due to "microvoid coalescence" being the mode of failure.
The most accurate way of evaluating K-R curve is taking presence of plasticity into account depending on the relative size of the plastic zone. For the case of negligible plasticity, the load vs displacement curve is obtained from the test and on each point the compliance is found. The compliance is reciprocal of the slope of the curve that will be followed if the specimen is unloaded at a certain point, which can be given as the ratio of displacement to load for LEFM. The compliance is used to determine the instantaneous crack length through the relationship given in the ASTM standard.
The stress intensity should be corrected by calculating an effective crack length. ASTM standard suggests two alternative approaches. The first method is named Irwin's plastic zone correction. Irwin's approach describes the effective crack length to be[18]
Irwin's approach leads to an iterative solution as K itself is a function of crack length.
The other method, namely the secant method, uses the compliance-crack length equation given by ASTM standard to calculate effective crack length from an effective compliance. Compliance at any point in Load vs displacement curve is essentially the reciprocal of the slope of the curve that ensues if the specimen is unloaded at that point. Now the unloading curve returns to the origin for linear elastic material but not for elastic plastic material as there is a permanent deformation. The effective compliance at a point for the elastic plastic case is taken as the slope of the line joining the point and origin (i.e the compliance if the material was an elastic one). This effective compliance is used to get an effective crack growth and the rest of the calculation follows the equation
The choice of plasticity correction is factored on the size of plastic zone. ASTM standard covering resistance curve suggests using Irwin's method is acceptable for small plastic zone and recommends using Secant method when crack-tip plasticity is more prominent. Also since the ASTM E 561 standard does not contain requirements on the specimen size or maximum allowable crack extension, thus the size independence of the resistance curve is not guaranteed. Few studies show that the size dependence is less detected in the experimental data for the Secant method.
Determination of JIC
Strain energy release rate per unit fracture surface area is calculated by J-integral method which is a contour path integral around the crack tip where the path begins and ends on either crack surfaces. J-toughness value signifies the resistance of the material in terms of amount of stress energy required for a crack to grow. JIC toughness value is measured for elastic-plastic materials. Now the single-valued JIC is determined as the toughness near the onset of the ductile crack extension (effect of strain hardening is not important). The test is performed with multiple specimen loading each of the specimen to various levels and unloading. This gives the crack mouth opening compliance which is to be used to get crack length with the help of relationships given in ASTM standard E 1820, which covers the J-integral testing.[19] Another way of measuring crack growth is to mark the specimen with heat tinting or fatigue cracking. The specimen is eventually broken apart and the crack extension is measured with the help of the marks.
The test thus performed yields several Load vs Crack Mouth Opening Displacement (CMOD) curve, which are used to calculate J as following:-
The linear elastic J is calculated using
and K is determined from where BN is the net thickness for side-grooved specimen and equal to B for not side-grooved specimen
The elastic plastic J is calculated using
Where =2 for SENB specimen
bo is initial ligament length given by the difference between width and initial crack length
APl is the plastic area under the load-displacement curve.
Specialized data reduction technique is used to get a provisional JQ. The value is accepted if the following criterion is met
Determination of tear resistance (Kahn tear test)
The tear test (e.g. Kahn tear test) provides a semi-quantitative measure of toughness in terms of tear resistance. This type of test requires a smaller specimen, and can, therefore, be used for a wider range of product forms. The tear test can also be used for very ductile aluminium alloys (e.g. 1100, 3003), where linear elastic fracture mechanics do not apply.
Standard test methods
A number of organizations publish standards related to fracture toughness measurements, namely ASTM, BSI, ISO, JSME.
- ASTM C1161 Test Method for Flexural Strength of Advanced Ceramics at Ambient Temperature
- ASTM E399 Test Method for Plane-strain Fracture Toughness of Metallic Materials
- ASTM E740 Practice for Fracture Testing with Surface-Crack Tension Specimens
- ASTM E1820 Standard Test Method for Measurement of Fracture Toughness
- ASTM E1823 Terminology Relating to Fatigue and Fracture Testing
- ISO 12135 Metallic materials — Unified method of test for the determination of quasistatic fracture toughness
- ISO 28079:2009, the Palmqvist method, used to determine the fracture toughness for cemented carbides.[20]
See also
References
- Suresh, S. (2004). Fatigue of Materials. Cambridge University Press. ISBN 978-0-521-57046-6.
- Kaufman, J. Gilbert (2015), Aluminum Alloy Database, Knovel, retrieved 1 August 2019
- ASM International Handbook Committee (1996), ASM Handbook, Volume 19 - Fatigue and Fracture, ASM International, p. 377
- Titanium Alloys - Ti6Al4V Grade 5, AZO Materials, 2000, retrieved 24 September 2014
- AR Boccaccini; S Atiq; DN Boccaccini; I Dlouhy; C Kaya (2005). "Fracture behaviour of mullite fibre reinforced-mullite matrix composites under quasi-static and ballistic impact loading". Composites Science and Technology. 65 (2): 325–333. doi:10.1016/j.compscitech.2004.08.002.
- J. Phalippou; T. Woignier; R. Rogier (1989). "Fracture toughness of silica aerogels". Journal de Physique Colloques. 50: C4–191. doi:10.1051/jphyscol:1989431.
- Wei, Robert (2010), Fracture Mechanics: Integration of Mechanics, Materials Science and Chemistry, Cambridge University Press, ASIN 052119489X
- Courtney, Thomas H. (2000). Mechanical behavior of materials. McGraw Hill. ISBN 9781577664253. OCLC 41932585.
- Padture, Nitin (12 April 2002). "Thermal Barrier Coatings for Gas-Turbine Engine Applications". Science. 296 (5566): 280–284. Bibcode:2002Sci...296..280P. doi:10.1126/science.1068609. PMID 11951028.
- Liang, Yiling (2010), The toughening mechanism in hybrid epoxy-silica-rubber nanocomposites, Lehigh University, p. 20, OCLC 591591884
- E08 Committee. "Test Method for Measurement of Fracture Toughness". doi:10.1520/e1820-20a. Cite journal requires
|journal=(help) - "Standard Terminology Relating to Fatigue Fracture Testing". www.astm.org. doi:10.1520/e1823-13. Retrieved 10 May 2019.
- "Standard Test Method for Plane-Strain Fracture Toughness of Metallic Materials". www.astm.org. doi:10.1520/e0399-90r97. Retrieved 10 May 2019.
- Andrews, WR; Shih, CF. "Thickness and Side-Groove Effects on J- and δ-Resistance Curves for A533-B Steel at 93C". www.astm.org: 426. doi:10.1520/stp35842s. Retrieved 10 May 2019.
- "Standard Test Method for Plane-Strain Fracture Toughness of Metallic Materials". www.astm.org. doi:10.1520/e0399-90r97. Retrieved 10 May 2019.
- "Stress Intensity Factors Compliances And Elastic Nu Factors For Six Test Geometries".
- "Standard Practice for R-Curve Determination". www.astm.org. doi:10.1520/e0561-98. Retrieved 10 May 2019.
- Liu, M.; et al. (2015). "An improved semi-analytical solution for stress at round-tip notches" (PDF). Engineering Fracture Mechanics. 149: 134–143. doi:10.1016/j.engfracmech.2015.10.004.
- "Standard Test Method for Measurement of Fracture Toughness". www.astm.org. doi:10.1520/e1820-01. Retrieved 10 May 2019.
- ISO 28079:2009, Palmqvist toughness test, Retrieved 22 January 2016
Further reading
- Anderson, T. L., Fracture Mechanics: Fundamentals and Applications (CRC Press, Boston 1995).
- Davidge, R. W., Mechanical Behavior of Ceramics (Cambridge University Press 1979).
- Knott, K. F., Fundamentals of Fracture Mechanics (1973).
- Suresh, S., Fatigue of Materials (Cambridge University Press 1998, 2nd edition).