Insensitive nuclei enhanced by polarization transfer

Insensitive nuclei enhancement by polarization transfer (INEPT) is a signal resolution enhancement method used in NMR spectroscopy. It involves the transfer of nuclear spin polarization from spins with large Boltzmann population differences to nuclear spins of interest with low Boltzmann population differences.[1] INEPT uses J-coupling for the polarization transfer in contrast to Nuclear Overhauser effect (NOE) which arises from dipolar cross-relaxation. This method of signal enhancement was introduced by Ray Freeman in 1979. Due to its usefulness in signal enhancement, pulse sequences used in heteronuclear NMR experiments often contain blocks of INEPT or INEPT-like sequences.

Background

The sensitivity of NMR signal detection depends on the gyromagnetic ratio (γ) of the nucleus. In general, the signal intensity produced from a nucleus with a gyromagnetic ratio of γ is proportional to γ3 because the magnetic moment, the Boltzmann populations, and the nuclear precession all increase in proportion to the gyromagnetic ratio γ. For example, the gyromagnetic ratio of 13C is 4 times lower than that of the proton, so the signal intensity it produces will be 64 times lower than one produced by a proton. However, noise also increases as the square root of the frequency, the sensitivity therefore becomes roughly proportional to γ5/2.[2] A 13C nucleus would be 32 times less sensitive than a proton, and 15N around 300 times less sensitive. Sensitivity enhancement techniques are therefore desirable when recording an NMR signal from an insensitive nucleus.

The sensitivity can be enhanced artificially by increasing the Boltzmann factors. One method may be through NOE, for example, for 13C signal, the signal-to-noise ratio can be improved three-fold when the attached protons are saturated. However, for NOE, a negative value of K, the ratio of gyromagnetic ratios of the nuclei, may result in a reduction in signal intensity. For 15N (Nitrogen-15 NMR spectroscopy) which has a negative gyromagnetic ratio, if the dipolar relaxation has to compete with other mechanisms, the observed 15N signal can be near zero.[2] Alternative methods are therefore necessary for nuclei with a negative gyromagnetic ratio, and one such method using the INEPT pulse sequence was proposed by Ray Freeman in 1979,[1] a method that became widely adopted.

Signal enhancement via the INEPT technique

The INEPT signal enhancement has two sources:

  • The spin population effect increases the signal by a factor of K = ratio of gyromagnetic ratios γI/γS of the nuclei, where γI and γS are the gyromagnetic ratio of the proton (the I spins) and the low-sensitivity nuclei (the S spins) respectively.
  • Nuclei with higher magnetogyric ratio generally relax more quickly. Since the rate at which the INEPT transfer that can be repeated is limited by the relaxation of these spins (rather than the low sensitivity spins), then the experiment can be repeated more frequently, increasing the signal-to-noise ratio.

As a result, INEPT can enhance the NMR signal by a factor larger than K, while the maximum enhancement via NOE is by a factor of 1+K/2.[1] Unlike NOE, no penalty is incurred by a negative gyromagnetic ratio in INEPT, it is therefore a useful method for enhancing the signal from nuclei with negative gyromagnetic ratio such as 15N or 29Si. The 15N signal may be enhanced by a factor of 10 via INEPT.[2]

Pulse sequence
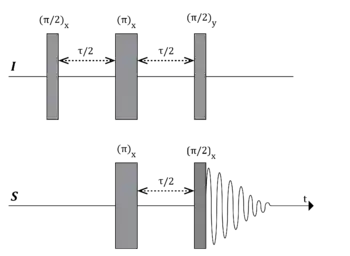
Graphical representation of the INEPT NMR pulse sequence. The thin bar denotes a 90° pulse, while the thick bar denotes a 180° pulse. INEPT is utilized often to improve 15N resolution because it can accommodate negative gyromagnetic ratios, increases Boltzmann polarization, and decreases T1 relaxation.[3]

The pulse sequence of INEPT, as represented in the diagram, can be read as a combination of spin echo and selective population inversion (SPI). The spin echo is a 90° pulse followed by a 180° separated by a time period τ and is applied on the proton, the sensitive nucleus (designated perhaps counter-intuitively as the I spin, with the insensitive nucleus the S spin, note however that the original paper on INEPT used the opposite designations).[1]

Spin Echo
90°I (X) — τ — 180°I (X)

The first 90° pulse flips the magnetization onto the +y axis and, due to the inhomogeneity of the field, the isochromats fan out at slightly different rate. After a time period, a 180° pulse is applied along the x axis, rotating the isochromats onto the -y axis. As individual isochromat still processes at the same rate as before, all the isochromats would converge and become refocused, thereby regenerating the signal, i.e. the echo. The chemical shifts are also refocused at the same time as the inhomogeneity, and this property allows the magnetization to be manipulated independent of the chemical shifts. The refocusing allows all the proton chemical shifts to undergo population inversion in the SPI step without its undesirable selectivity.

Selective Population Inversion
180°S — τ — 90°I (Y), 90°S — Acquisition

As shown in the diagram, a 180° pulse is applied on the insensitive nucleus simultaneously with the 180° pulse on the proton. This is the population inversion part of the scheme, where a further 90° pulse after a time period on both the sensitive and insensitive nuclei rotate the magnetization onto the z-axis. This has the effect of producing an antiphase alignment of magnetization on the z axis, an important step during which the polarization is transferred from the sensitive nucleus to the insensitive one.[2]

Variations

There are a number of variations of the experiments, for example, a refocusing step or an extra 90° 1H pulse may be added, and there are also reverse INEPT pulse sequences.[4]

References
  1. Gareth A. Morris, Ray Freeman (1979). "Enhancement of Nuclear Magnetic Resonance Signals by Polarization Transfer". Journal of the American Chemical Society. 101 (3): 760–762. doi:10.1021/ja00497a058.
  2. Ray Freeman (1987). A Handbook of Nuclear Magnetic Resonance (2 ed.). Longman. p. 178. ISBN 0-582-25184-2.
  3. M H Levitt (2008). Spin Dynamics. John Wiley & Sons Ltd. ISBN 0470511176.
  4. "ID Experiments: INEPT".
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.