Kibble balance

A Kibble balance is an electromechanical measuring instrument that measures the weight of a test object very precisely by the electric current and voltage needed to produce a compensating force. It is a metrological instrument that can realize the definition of the kilogram unit of mass based on fundamental constants.[1][2]

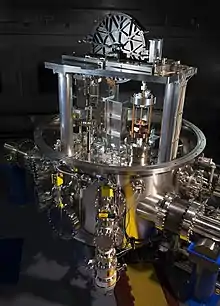
The NIST-4 Kibble balance, which began full operation in early 2015, measured Planck's constant to within 13 parts per billion in 2017, which was accurate enough to assist with the 2019 redefinition of the kilogram.

It was originally called the watt balance because the weight of the test mass is proportional to the product of current and voltage, which is measured in watts. In June 2016, two months after the death of its inventor, Bryan Kibble, metrologists of the Consultative Committee for Units of the International Committee for Weights and Measures agreed to rename the device in his honor.[3][4]

Prior to 2019, the definition of the kilogram was based on a physical object known as the International Prototype of the Kilogram (IPK). After considering alternatives, in 2013 the General Conference on Weights and Measures (CGPM) agreed on accuracy criteria for replacing this definition with one based on the use of a Kibble balance. After these criteria had been achieved, the CGPM voted unanimously on November 16, 2018 to change the definition of the kilogram and several other units, effective May 20, 2019, to coincide with World Metrology Day.[3][5][6][7][8]

Design
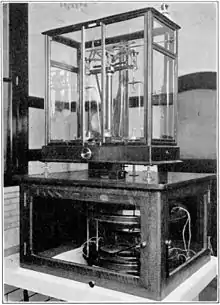
Precision Ampere balance at the US National Bureau of Standards (now NIST) in 1927. The current coils are visible under the balance, attached to the right balance arm. The Kibble balance is a development of the Ampere balance.

The Kibble balance is a more accurate version of the ampere balance, an early current measuring instrument in which the force between two current-carrying coils of wire is measured and then used to calculate the magnitude of the current. The Kibble balance operates in the opposite sense; the current in the coils is measured using the definition of the Planck constant to "measure mass without recourse to the IPK or any physical object."[9] The balance determines the weight of the object; then the mass is calculated by accurately measuring the local Earth's gravity (the net acceleration combining gravitational and centrifugal effects) with a gravimeter. Thus the mass of the object is defined in terms of a current and a voltage—an "electronic kilogram."

Origin

The principle that is used in the Kibble balance was proposed by Bryan Kibble of the UK National Physical Laboratory (NPL) in 1975 for measurement of the gyromagnetic ratio.[10]

The main weakness of the ampere balance method is that the result depends on the accuracy with which the dimensions of the coils are measured. The Kibble balance uses an extra calibration step to cancel the effect of the geometry of the coils, removing the main source of uncertainty. This extra step involves moving the force coil through a known magnetic flux at a known speed. This step was first performed in 1990.[11]

The Kibble balance originating from the National Physical Laboratory was transferred to the National Research Council of Canada (NRC) in 2009, where scientists from the two labs continued to refine the instrument.[12] In 2014, NRC researchers published the most accurate measurement of the Planck constant at that time, with a relative uncertainty of 1.8×10−8.[13] A final paper by NRC researchers was published in May 2017, presenting a measurement of Planck's constant with an uncertainty of only 9.1 parts per billion, the measurement with the least uncertainty to that date.[14] Other Kibble balance experiments are conducted in the US National Institute of Standards and Technology (NIST), the Swiss Federal Office of Metrology (METAS) in Berne, the International Bureau of Weights and Measures (BIPM) near Paris and Laboratoire national de métrologie et d’essais (LNE) in Trappes, France.[15]

Principle

A conducting wire of length L that carries an electric current I perpendicular to a magnetic field of strength B experiences a Lorentz force equal to the product of these variables. In the Kibble balance, the current is varied so that this force counteracts the weight w of a mass m to be measured. This principle is derived from the ampere balance. w is given by the mass m multiplied by the local gravitational acceleration g. Thus,

The Kibble balance avoids the problems of measuring B and L in a second calibration step. The same wire (in practice, a coil) is moved through the same magnetic field at a known speed v. By Faraday's law of induction, a potential difference U is generated across the ends of the wire, which equals BLv. Thus

The unknown product BL can be eliminated from the equations to give

With U, I, g, and v accurately measured, this gives an accurate value for m. Both sides of the equation have the dimensions of power, measured in watts in the International System of Units; hence the original name "watt balance".

Implementation
Weighing mode
Moving mode

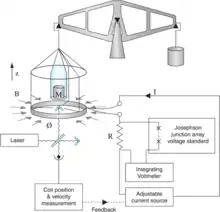
The Kibble balance is constructed so that the mass to be measured and the wire coil are suspended from one side of a balance scale, with a counterbalance mass on the other side. The system operates by alternating between two modes: "weighing" and "moving". The entire mechanical subsystem operates in a vacuum chamber to remove the effects of air buoyancy.[16]

While "weighing", the system measures the "I" component and the "v" component. The system controls the current in the coil to pull the coil through a magnetic field at a constant velocity "v". Coil position and velocity measurement circuitry uses an interferometer together with a precision clock input to determine the velocity and control the current needed to maintain it. The required current is measured, using an ammeter comprising a Josephson junction voltage standard and an integrating voltmeter.

While "moving", the system measures the "U" component. The system ceases to provide current to the coil. This allows the counterbalance to pull the coil (and mass) upward through the magnetic field, which causes a voltage difference across the coil. The velocity measurement circuitry measures the speed of movement of the coil. This voltage is measured, using the same voltage standard and integrating voltmeter.

A typical Kibble balance measures U, I, and v, but does not measure the local gravitational acceleration "g", because "g" does not vary rapidly with time. Instead, "g" is measured in the same laboratory using a highly accurate and precise gravimeter. In addition, the balance depends on a highly accurate and precise frequency reference such as an atomic clock to compute voltage and amperage. Thus, the precision and accuracy of the mass measurement depends on the Kibble balance, the gravimeter, and the clock.

Like the early atomic clocks, the early Kibble balances were one-of-a-kind experimental devices and were large, expensive, and delicate. As of 2019, work is underway to produce standardized devices at prices that permit use in any metrology laboratory that requires high-precision measurement of mass.[17]

As well as large Kibble balances, microfabricated or MEMS watt balances (now called Kibble balances) have been demonstrated[18] since around 2003. These are fabricated on single silicon dies similar to those used in microelectronics and accelerometers, and are capable of measuring small forces in the nanonewton to micronewton range traceably to the SI-defined physical constants via electrical and optical measurements. Due to their small scale, MEMS Kibble balances typically use electrostatic rather than the inductive forces used in larger instruments. Lateral and torsional[19] variants have also been demonstrated, with the main application (as of 2019) being in the calibration of the Atomic Force Microscope.

Measurements

Accurate measurements of electric current and potential difference are made in conventional electrical units (rather than SI units), which are based on fixed "conventional values" of the Josephson constant and the von Klitzing constant, and respectively. The current Kibble balance experiments are equivalent to measuring the value of the conventional watt in SI units. From the definition of the conventional watt, this is equivalent to measuring the value of the product KJ2RK in SI units instead of its fixed value in conventional electrical units:

The importance of such measurements is that they are also a direct measurement of the Planck constant h:

The principle of the electronic kilogram relies on the value of the Planck constant, which is as of 2019 an exact value. This is similar to the metre being defined by the speed of light. With the constant defined exactly, the Kibble balance is not an instrument to measure the Planck constant, but is instead an instrument to measure mass:

See also

References
  1. Robinson, Ian A.; Schlamminger, Stephan (2016). "The watt or Kibble balance: A technique for implementing the new SI definition of the unit of mass". Metrologia. 53 (5): A46–A74. doi:10.1088/0026-1394/53/5/A46.
  2. Palmer, Jason (2011-01-26). "Curbing the kilogram's weight-loss programme". BBC News. BBC News. Retrieved 2011-02-16.
  3. "The Kibble Balance". Education. UK National Physical Laboratory website. 2016. Retrieved 15 May 2017.
  4. Consultative Committee for Units (CCU), Report of the 22nd meeting (15-16 June 2016), pp. 32-32, 35
  5. Cho, Adrian (2017). "Plot to redefine the kilogram nears climax". Science. 356 (6339): 670–671. doi:10.1126/science.356.6339.670. PMID 28522473.
  6. Milton, Martin (14 November 2016). "Highlights in the work of the BIPM in 2016" (PDF). p. 10. Archived from the original (PDF) on 1 September 2017. Retrieved 1 September 2017.
  7. Decision CIPM/105-13 (October 2016)
  8. Materese, Robin (2018-11-16). "Historic Vote Ties Kilogram and Other Units to Natural Constants". NIST. Retrieved 2018-11-16.
  9. Materese, Robin (2018-05-14). "Kilogram: The Kibble Balance". NIST. Retrieved 2018-11-22.
  10. Kibble, B. P. (1976). "A Measurement of the Gyromagnetic Ratio of the Proton by the Strong Field Method". Atomic Masses and Fundamental Constants 5. pp. 545–551. doi:10.1007/978-1-4684-2682-3_80. ISBN 978-1-4684-2684-7.
  11. Kibble, B. P.; Robinson, I. A.; Belliss, J. H. (1990). "A Realization of the SI Watt by the NPL Moving-coil Balance". Metrologia. 27 (4): 173–192. doi:10.1088/0026-1394/27/4/002.
  12. "Kibble balances : Research : Mass & Force : Science + Technology : National Physical Laboratory". www.npl.co.uk.
  13. Sanchez, C. A.; Wood, B. M.; Green, R. G.; Liard, J. O.; Inglis, D. (2014). "A determination of Planck's constant using the NRC watt balance". Metrologia. 51 (2): S5–S14. doi:10.1088/0026-1394/51/2/S5.
  14. Wood, B. M.; Sanchez, C. A.; Green, R. G.; Liard, J. O. (2017). "A summary of the Planck constant determinations using the NRC Kibble balance". Metrologia. 54 (3): 399–409. doi:10.1088/1681-7575/aa70bf.
  15. Mohr, Peter J.; Taylor, Barry N.; Newell, David B. (2008). "CODATA Recommended Values of the Fundamental Physical Constants: 2006" (PDF). Reviews of Modern Physics. 80 (2): 633–730. arXiv:0801.0028. Bibcode:2008RvMP...80..633M. doi:10.1103/RevModPhys.80.633. Archived from the original (PDF) on 2017-10-01.
  16. Robinson, Ian; Schlamminger, Stephan (2016). "The watt or Kibble balance: A technique for implementing the new SI definition of the unit of mass". Metrologia. 53 (5): A46–A74. doi:10.1088/0026-1394/53/5/A46.
  17. Conover, Emily (June 3, 2019). "This tabletop device turns the quantum definition of a kilogram into a real mass". ScienceNews.
  18. Cumpson, Peter J.; Hedley, John (2003). "Accurate analytical measurements in the atomic force microscope: a microfabricated spring constant standard potentially traceable to the SI". Nanotechnology. 14 (12): 1279–1288. doi:10.1088/0957-4484/14/12/009.
  19. Portoles, Jose F.; Cumpson, Peter J. (2013). "A compact torsional reference device for easy, accurate and traceable AFM piconewton calibration". Nanotechnology. 24 (33): 335706. doi:10.1088/0957-4484/24/33/335706.


This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.