Monogenic semigroup

In mathematics, a monogenic semigroup is a semigroup generated by a single element.[1] Monogenic semigroups are also called cyclic semigroups.[2]

Monogenic semigroup of order 9 and period 6. Numbers are exponents of the generator a; arrows indicate multiplication by a.

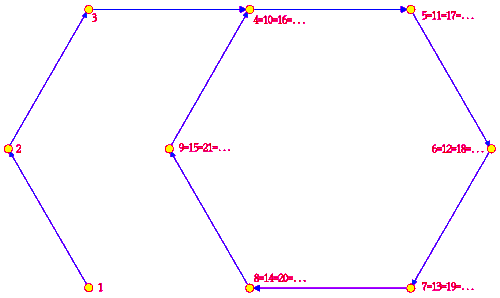
Structure

The monogenic semigroup generated by the singleton set {a} is denoted by ${\displaystyle \langle a\rangle }$. The set of elements of ${\displaystyle \langle a\rangle }$ is {a, a², a³, ...}. There are two possibilities for the monogenic semigroup ${\displaystyle \langle a\rangle }$:

• a ^m = a ⁿ ⇒ m = n.
• There exist m ≠ n such that a ^m = a ⁿ.

In the former case ${\displaystyle \langle a\rangle }$ is isomorphic to the semigroup ( {1, 2, ...}, + ) of natural numbers under addition. In such a case, ${\displaystyle \langle a\rangle }$ is an infinite monogenic semigroup and the element a is said to have infinite order. It is sometimes called the free monogenic semigroup because it is also a free semigroup with one generator.

In the latter case let m be the smallest positive integer such that a ^m = a ^x for some positive integer x ≠ m, and let r be smallest positive integer such that a ^m = a ^{m + r}. The positive integer m is referred to as the index and the positive integer r as the period of the monogenic semigroup ${\displaystyle \langle a\rangle }$. The order of a is defined as m+r-1. The period and the index satisfy the following properties:

• a ^m = a ^{m + r}
• a ^{m + x} = a ^{m + y} if and only if m + x ≡ m + y ( mod r )
• ${\displaystyle \langle a\rangle }$ = {a, a², ... , a ^{m + r − 1}}
• K_a = {a^m, a ^{m + 1}, ... , a ^{m + r − 1}} is a cyclic subgroup and also an ideal of ${\displaystyle \langle a\rangle }$. It is called the kernel of a and it is the minimal ideal of the monogenic semigroup ${\displaystyle \langle a\rangle }$.[3][4]

The pair ( m, r ) of positive integers determine the structure of monogenic semigroups. For every pair ( m, r ) of positive integers, there does exist a monogenic semigroup having index m and period r. The monogenic semigroup having index m and period r is denoted by M ( m, r ). The monogenic semigroup M ( 1, r ) is the cyclic group of order r.

The results in this section actually hold for any element a of an arbitrary semigroup and the monogenic subsemigroup ${\displaystyle \langle a\rangle }$ it generates.

Related notions

A related notion is that of periodic semigroup (also called torsion semigroup), in which every element has finite order (or, equivalently, in which every mongenic subsemigroup is finite). A more general class is that of quasi-periodic semigroups (aka group-bound semigroups or epigroups) in which every element of the semigroup has a power that lies in a subgroup.[5][6]

An aperiodic semigroup is one in which every monogenic subsemigroup has a period of 1.

See also

• Cycle detection, the problem of finding the parameters of a finite monogenic semigroup using a bounded amount of storage space
• Special classes of semigroups

References

1. Howie, J M (1976). An Introduction to Semigroup Theory. L.M.S. Monographs. 7. Academic Press. pp. 7–11. ISBN 0-12-356950-8.
2. A H Clifford; G B Preston (1961). The Algebraic Theory of Semigroups Vol.I. Mathematical Surveys. 7. American Mathematical Society. pp. 19–20. ISBN 978-0821802724.
3. http://www.encyclopediaofmath.org/index.php/Kernel_of_a_semi-group
4. http://www.encyclopediaofmath.org/index.php/Minimal_ideal
5. http://www.encyclopediaofmath.org/index.php/Periodic_semi-group
6. Peter M. Higgins (1992). Techniques of semigroup theory. Oxford University Press. p. 4. ISBN 978-0-19-853577-5.