Pseudo-deltoidal icositetrahedron

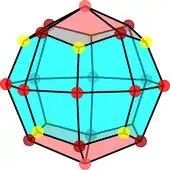
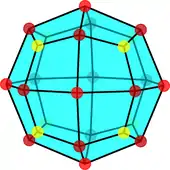
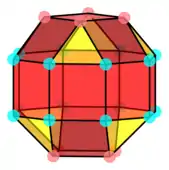
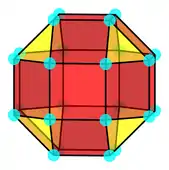
The pseudo-deltoidal icositetrahedron is a convex polyhedron with 24 kites as its faces. It is the dual of the elongated square gyrobicupola (also known as the pseudorhombicuboctahedron).

Pseudo-deltoidal icositetrahedron
(see [[:File:Pseudodeltoidal icositetrahedron.stl|3D model)
Type	Johnson solid dual, Pseudo-uniform polyhedron dual
Faces	24 kites
Edges	48
Vertices	26
Vertex configuration	(2) 4.4.4 (8+8+2) 4.4.4.4
Symmetry group	Dihedral (D4d)
Dual polyhedron	Elongated square gyrobicupola
Properties	convex
Net	(click to enlarge)

It is similar to the deltoidal icositetrahedron, but has a twist, similar to the relationship between the pseudorhombicuboctahedron and the rhombicuboctahedron. As the pseudorhombicuboctahedron is not truly vertex-transitive, the pseudo-deltoidal icositetrahedron is not truly face-transitive, with its faces in two different symmetry orbits (three if one only considers rotational symmetries); however, since the pseudorhombicuboctahedron has a singular vertex figure, the pseudo-deltoidal icositetrahedron has only one type of face (monohedral).

Pseudo- and actual deltoidal icositetrahedron Pseudo- and actual rhombicuboctahedron

Pseudo- and actual deltoidal icositetrahedron Pseudo- and actual great deltoidal icositetrahedron

Die

External links

• George Hart: pseudo-rhombicuboctahedra