Shear force

In solid mechanics, shearing forces are unaligned forces pushing one part of a body in one specific direction, and another part of the body in the opposite direction. When the forces are colinear (aligned into each other), they are called compression forces. An example is a deck of cards being pushed one way on the top, and the other at the bottom, causing the cards to slide. Another example is when wind blows at the side of a peaked roof of a house - the side walls experience a force at their top pushing in the direction of the wind, and their bottom in the opposite direction, from the ground or foundation. William A. Nash defines shear force in terms of planes: "If a plane is passed through a body, a force acting along this plane is called a shear force or shearing force."[1]

Shearing forces push in one direction at the top, and the opposite direction at the bottom, causing shearing deformation.
A crack or tear may develop in a body from parallel shearing forces pushing in opposite directions at different points of the body. If the forces were aligned and aimed straight into each other, they would pinch or compress the body, rather than tear or crack it.
Further information: Shear stress

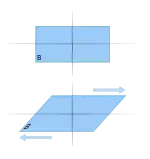
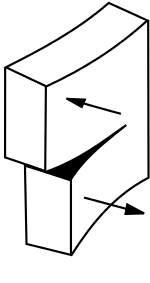
Shear force of steel and bolts

Here follows a short example of how to work out the shear force of a piece of steel. The factor of 0.6 used to change from tensile to shear force could vary from 0.58–0.62 and will depend on application.

Steel called EN8 bright has a tensile strength of 800 MPa and mild steel has a tensile strength of 400 MPa.

To work out the force to shear a 25 mm diameter round steel EN8 bright;

Area of the 25 mm round steel in mm2 = (12.52)(π) ≈ 490.8 mm2
0.8 kN/mm2 × 490.8 mm2 = 392.64 kN ≈ 40 tonne-force
40 tonne-force × 0.6 (to change force from tensile to shear) = 24 tonne-force

When working with a riveted or tensioned bolted joint, the strength comes from friction between the materials bolted together. Bolts are correctly torqued to maintain the friction. The shear force only becomes relevant when the bolts are not torqued.

A bolt with property class 12.9 has a tensile strength of 1200 MPa (1 MPa = 1 N/mm2) or 1.2 kN/mm2 and the yield strength is 0.90 times tensile strength, 1080 MPa in this case.

A bolt with property class 4.6 has a tensile strength of 400 MPa (1 MPa = 1 N/mm2) or 0.4 kN/mm2 and yield strength is 0.60 times tensile strength, 240 MPa in this case.

In case of fasteners, proof load is specified as it gives a real-life picture about the characteristics of the bolt.

See also

References
  1. William A. Nash (1 July 1998). Schaum's Outline of Theory and Problems of Strength of Materials. McGraw-Hill Professional. p. 82. ISBN 978-0-07-046617-3. Retrieved 20 May 2012.
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.