Great dodecahedron
In geometry, the great dodecahedron is a Kepler–Poinsot polyhedron, with Schläfli symbol {5,5/2} and Coxeter–Dynkin diagram of ![]()
![]()
![]()
![]()
![]()
![]()
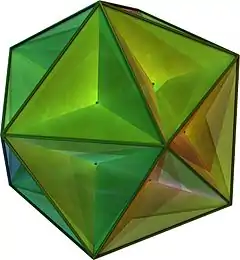
![]() . It is one of four nonconvex regular polyhedra. It is composed of 12 pentagonal faces (six pairs of parallel pentagons), with five pentagons meeting at each vertex, intersecting each other making a pentagrammic path.
. It is one of four nonconvex regular polyhedra. It is composed of 12 pentagonal faces (six pairs of parallel pentagons), with five pentagons meeting at each vertex, intersecting each other making a pentagrammic path.
| Great dodecahedron | |
|---|---|
 | |
| Type | Kepler–Poinsot polyhedron |
| Stellation core | regular dodecahedron |
| Elements | F = 12, E = 30 V = 12 (χ = -6) |
| Faces by sides | 12{5} |
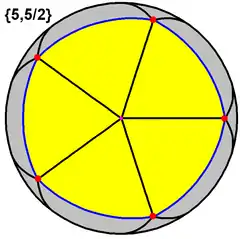
| Schläfli symbol | {5,5⁄2} |
| Face configuration | V(5⁄2)5 |
| Wythoff symbol | 2 5 |
| Coxeter diagram | |
| Symmetry group | Ih, H3, [5,3], (*532) |
| References | U35, C44, W21 |
| Properties | Regular nonconvex |
 (55)/2 (Vertex figure) |
 Small stellated dodecahedron (dual polyhedron) |

The discovery of the great dodecahedron is sometimes credited to Louis Poinsot in 1810, though there is a drawing of something very similar to a great dodecahedron in the 1568 book Perspectiva Corporum Regularium by Wenzel Jamnitzer.
The great dodecahedron can be constructed analogously to the pentagram, its two-dimensional analogue, via the extension of the (n-1)-D pentagonal polytope faces of the core nD polytope (pentagons for the great dodecahedron, and line segments for the pentagram) until the figure again closes.
Images
| Transparent model | Spherical tiling |
|---|---|
 (With animation) |
 This polyhedron represents a spherical tiling with a density of 3. (One spherical pentagon face is shown above in yellow) |
| Net | Stellation |
 × 20 × 20Net for surface geometry; twenty isosceles triangular pyramids, arranged like the faces of an icosahedron |
 It can also be constructed as the second of three stellations of the dodecahedron, and referenced as Wenninger model [W21]. |
Related polyhedra

It shares the same edge arrangement as the convex regular icosahedron; the compound with both is the small complex icosidodecahedron.
If only the visible surface is considered, it has the same topology as a triakis icosahedron with concave pyramids rather than convex ones. The excavated dodecahedron can be seen as the same process applied to a regular dodecahedron, although this result is not regular.
A truncation process applied to the great dodecahedron produces a series of nonconvex uniform polyhedra. Truncating edges down to points produces the dodecadodecahedron as a rectified great dodecahedron. The process completes as a birectification, reducing the original faces down to points, and producing the small stellated dodecahedron.
| Stellations of the dodecahedron | ||||||
| Platonic solid | Kepler–Poinsot solids | |||||
| Dodecahedron | Small stellated dodecahedron | Great dodecahedron | Great stellated dodecahedron | |||
|---|---|---|---|---|---|---|
 |
 |
 |
 | |||
 |
 |
 |
 | |||
| Name | Small stellated dodecahedron | Dodecadodecahedron | Truncated great dodecahedron |
Great dodecahedron |
|---|---|---|---|---|
| Coxeter-Dynkin diagram |
||||
| Picture |  |
 |
 |
 |
Usage
- This shape was the basis for the Rubik's Cube-like Alexander's Star puzzle.
- The great dodecahedron provides an easy mnemonic for the binary Golay code[1]
References
-
- Baez, John "Golay code," Visual Insight, December 1, 2015.