Radian per second
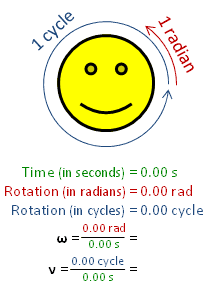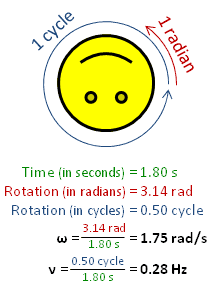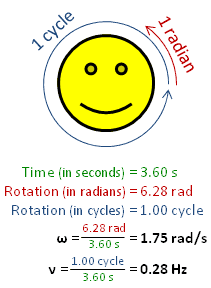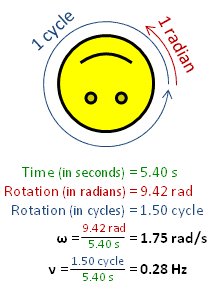
The radian per second (symbol: rad⋅s−1 or rad/s) is the SI unit of angular velocity, commonly denoted by the Greek letter ω (omega). The radian per second is also the SI unit of angular frequency. The radian per second is defined as the change in the orientation of an object, in radians, every second.
Angular frequency ω (Ordinary) frequency 2π radians per second exactly 1 hertz (Hz) 1 radian per second approximately 0.159155 Hz 1 radian per second approximately 57.29578 degrees per second 1 radian per second approximately 9.5493 revolutions per minute (rpm) 0.1047 radians per second approximately 1 rpm
| Radian per second | |
|---|---|
 | |
| General information | |
| Unit system | SI derived unit |
| Unit of | rotational speed |
| Symbol | rad/s or rad⋅s−1 |
Since the radian is a dimensionless unit in the SI, the radian per second is dimensionally equivalent to the hertz—both are defined as s−1. This may lead to confusion between the quantities angular frequency ω and frequency ν.[1][2][3][4]
A use of the unit radian per second is in calculation of the power transmitted by a shaft. In the International System of Units, widely used in physics and engineering, the power p is equal to the rotational speed ω (in radians per second) multiplied by the torque τ applied to the shaft, in newton-metres. Thus, p = ω ⋅ τ, and the unit is the watt, with no numerical coefficient needed. In other systems, an additional factor may be necessary. For example, if one multiplies angular velocity in revolutions per minute (rpm) by the torque in pound-feet, then a factor is needed to convert the result to units of horsepower.
An angular frequency, ω = 1 rad/s, corresponds to an ordinary frequency, ν = 1/(2π) Hz ≈ 0.159 Hz, which corresponds to a frequency of rotation of 60/(2π) rpm ≈ 9.55 rpm. In other words 6 radians is about 360 degrees.
References
- Mohr, J. C.; Phillips, W. D. (2015). "Dimensionless Units in the SI". Metrologia. 52 (1): 40–47. arXiv:1409.2794. Bibcode:2015Metro..52...40M. doi:10.1088/0026-1394/52/1/40.
- Mills, I. M. (2016). "On the units radian and cycle for the quantity plane angle". Metrologia. 53 (3): 991–997. Bibcode:2016Metro..53..991M. doi:10.1088/0026-1394/53/3/991.
- "SI units need reform to avoid confusion". Editorial. Nature. 548 (7666): 135. 7 August 2011. doi:10.1038/548135b. PMID 28796224.
- P. R. Bunker; I. M. Mills; Per Jensen (2019). "The Planck constant and its units". J Quant Spectrosc Radiat Transfer. 237: 106594. doi:10.1016/j.jqsrt.2019.106594.