Refinement (category theory)
In category theory and related fields of mathematics, a refinement is a construction that generalizes the operations of "interior enrichment", like bornologification or saturation of a locally convex space. A dual construction is called envelope.
Definition
Suppose is a category, an object in , and and two classes of morphisms in . The definition[1] of a refinement of in the class by means of the class consists of two steps.
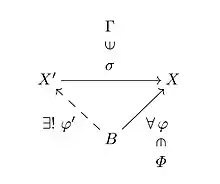
- A morphism in is called an enrichment of the object in the class of morphisms by means of the class of morphisms , if , and for any morphism from the class there exists a unique morphism in such that .
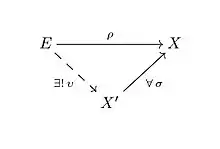
- An enrichment of the object in the class of morphisms by means of the class of morphisms is called a refinement of in by means of , if for any other enrichment (of in by means of ) there is a unique morphism in such that . The object is also called a refinement of in by means of .
Notations:
In a special case when is a class of all morphisms whose ranges belong to a given class of objects in it is convenient to replace with in the notations (and in the terms):
Similarly, if is a class of all morphisms whose ranges belong to a given class of objects in it is convenient to replace with in the notations (and in the terms):
For example, one can speak about a refinement of in the class of objects by means of the class of objects :
Examples
- The bornologification[2][3] of a locally convex space is a refinement of in the category of locally convex spaces by means of the subcategory of normed spaces:
- The saturation[4][3] of a pseudocomplete[5] locally convex space is a refinement in the category of locally convex spaces by means of the subcategory of the Smith spaces:
See also
Notes
- Akbarov 2016, p. 52.
- Kriegl & Michor 1997, p. 35.
- Akbarov 2016, p. 57.
- Akbarov 2003, p. 194.
- A topological vector space is said to be pseudocomplete if each totally bounded Cauchy net in converges.
References
- Kriegl, A.; Michor, P.W. (1997). The convenient setting of global analysis. Providence, Rhode Island: American Mathematical Society. ISBN 0-8218-0780-3.CS1 maint: ref=harv (link)
- Akbarov, S.S. (2003). "Pontryagin duality in the theory of topological vector spaces and in topological algebra". Journal of Mathematical Sciences. 113 (2): 179–349. doi:10.1023/A:1020929201133. S2CID 115297067.CS1 maint: ref=harv (link)
- Akbarov, S.S. (2016). "Envelopes and refinements in categories, with applications to functional analysis". Dissertationes Mathematicae. 513: 1–188. arXiv:1110.2013. doi:10.4064/dm702-12-2015. S2CID 118895911.CS1 maint: ref=harv (link)
