Catalan solid

In mathematics, a Catalan solid, or Archimedean dual, is a dual polyhedron to an Archimedean solid. There are 13 Catalan solids. They are named for the Belgian mathematician, Eugène Catalan, who first described them in 1865.

Triakis tetrahedron, pentagonal icositetrahedron and disdyakis triacontahedron.

The solids above (dark) shown together with their duals (light). The visible parts of the Catalan solids are regular pyramids.

A rhombic dodecahedron with its face configuration.

The Catalan solids are all convex. They are face-transitive but not vertex-transitive. This is because the dual Archimedean solids are vertex-transitive and not face-transitive. Note that unlike Platonic solids and Archimedean solids, the faces of Catalan solids are not regular polygons. However, the vertex figures of Catalan solids are regular, and they have constant dihedral angles. Being face-transitive, Catalan solids are isohedra.

Additionally, two of the Catalan solids are edge-transitive: the rhombic dodecahedron and the rhombic triacontahedron. These are the duals of the two quasi-regular Archimedean solids.

Just as prisms and antiprisms are generally not considered Archimedean solids, so bipyramids and trapezohedra are generally not considered Catalan solids, despite being face-transitive.

Two of the Catalan solids are chiral: the pentagonal icositetrahedron and the pentagonal hexecontahedron, dual to the chiral snub cube and snub dodecahedron. These each come in two enantiomorphs. Not counting the enantiomorphs, bipyramids, and trapezohedra, there are a total of 13 Catalan solids.

n	Archimedean solid	Catalan solid
1	truncated tetrahedron	triakis tetrahedron
2	truncated cube	triakis octahedron
3	truncated cuboctahedron	disdyakis dodecahedron
4	truncated octahedron	tetrakis hexahedron
5	truncated dodecahedron	triakis icosahedron
6	truncated icosidodecahedron	disdyakis triacontahedron
7	truncated icosahedron	pentakis dodecahedron
8	cuboctahedron	rhombic dodecahedron
9	icosidodecahedron	rhombic triacontahedron
10	rhombicuboctahedron	deltoidal icositetrahedron
11	rhombicosidodecahedron	deltoidal hexecontahedron
12	snub cube	pentagonal icositetrahedron
13	snub dodecahedron	pentagonal hexecontahedron

Symmetry

The Catalan solids, along with their dual Archimedean solids, can be grouped in those with tetrahedral, octahedral and icosahedral symmetry. For both octahedral and icosahedral symmetry there are six forms. The only Catalan solid with genuine tetrahedral symmetry is the triakis tetrahedron (dual of the truncated tetrahedron). Rhombic dodecahedron and tetrakis hexahedron have octahedral symmetry, but they can be colored to have only tetrahedral symmetry. Rectification and snub also exist with tetrahedral symmetry, but they are Platonic instead of Archimedean, so their duals are Platonic instead of Catalan. (They are shown with brown background in the table below.)

Tetrahedral symmetry

Archimedean (Platonic)
Catalan (Platonic)

Octahedral symmetry

Archimedean
Catalan

Icosahedral symmetry

Archimedean
Catalan

List

Name (Dual name) Conway name	Pictures	Orthogonal wireframes	Face polygon	Face angles (°)	Dihedral angle (°)	Faces	Edges	Vert	Sym.
triakis tetrahedron (truncated tetrahedron) "kT"			Isosceles V3.6.6	112.885 33.557 33.557	129.521	12	18	8	Td
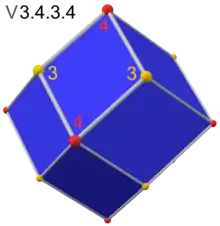
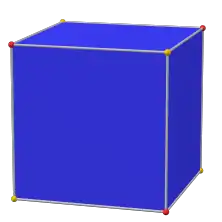
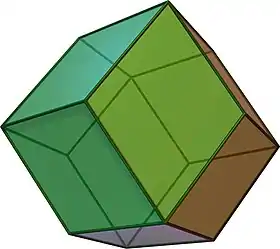
rhombic dodecahedron (cuboctahedron) "jC"			Rhombus V3.4.3.4	70.529 109.471 70.529 109.471	120	12	24	14	Oh
triakis octahedron (truncated cube) "kO"			Isosceles V3.8.8	117.201 31.400 31.400	147.350	24	36	14	Oh
tetrakis hexahedron (truncated octahedron) "kC"			Isosceles V4.6.6	83.621 48.190 48.190	143.130	24	36	14	Oh
deltoidal icositetrahedron (rhombicuboctahedron) "oC"			Kite V3.4.4.4	81.579 81.579 81.579 115.263	138.118	24	48	26	Oh
disdyakis dodecahedron (truncated cuboctahedron) "mC"			Scalene V4.6.8	87.202 55.025 37.773	155.082	48	72	26	Oh
pentagonal icositetrahedron (snub cube) "gC"			Pentagon V3.3.3.3.4	114.812 114.812 114.812 114.812 80.752	136.309	24	60	38	O
rhombic triacontahedron (icosidodecahedron) "jD"			Rhombus V3.5.3.5	63.435 116.565 63.435 116.565	144	30	60	32	Ih
triakis icosahedron (truncated dodecahedron) "kI"			Isosceles V3.10.10	119.039 30.480 30.480	160.613	60	90	32	Ih
pentakis dodecahedron (truncated icosahedron) "kD"			Isosceles V5.6.6	68.619 55.691 55.691	156.719	60	90	32	Ih
deltoidal hexecontahedron (rhombicosidodecahedron) "oD"			Kite V3.4.5.4	86.974 67.783 86.974 118.269	154.121	60	120	62	Ih
disdyakis triacontahedron (truncated icosidodecahedron) "mD"			Scalene V4.6.10	88.992 58.238 32.770	164.888	120	180	62	Ih
pentagonal hexecontahedron (snub dodecahedron) "gD"			Pentagon V3.3.3.3.5	118.137 118.137 118.137 118.137 67.454	153.179	60	150	92	I

Geometry

All dihedral angles of a Catalan solid are equal. Denoting their value by ${\displaystyle \theta }$ , and denoting the face angle at the vertices where ${\displaystyle p}$ faces meet by ${\displaystyle \alpha _{p}}$, we have

${\displaystyle \sin(\theta /2)=\cos(\pi /p)/\cos(\alpha _{p}/2)}$.

This can be used to compute ${\displaystyle \theta }$ and ${\displaystyle \alpha _{p}}$, ${\displaystyle \alpha _{q}}$, ... , from ${\displaystyle p}$, ${\displaystyle q}$ ... only.

Triangular faces

Of the 13 Catalan solids, 7 have triangular faces. These are of the form Vp.q.r, where p, q and r take their values among 3, 4, 5, 6, 8 and 10. The angles ${\displaystyle \alpha _{p}}$, ${\displaystyle \alpha _{q}}$ and ${\displaystyle \alpha _{r}}$ can be computed in the following way. Put ${\displaystyle a=4\cos ^{2}(\pi /p)}$, ${\displaystyle b=4\cos ^{2}(\pi /q)}$, ${\displaystyle c=4\cos ^{2}(\pi /r)}$ and put

${\displaystyle S=-a^{2}-b^{2}-c^{2}+2ab+2bc+2ca}$.

Then

${\displaystyle \cos(\alpha _{p})={\frac {S}{2bc}}-1}$,

${\displaystyle \sin(\alpha _{p}/2)={\frac {-a+b+c}{2{\sqrt {bc}}}}}$.

For ${\displaystyle \alpha _{q}}$ and ${\displaystyle \alpha _{r}}$ the expressions are similar of course. The dihedral angle ${\displaystyle \theta }$ can be computed from

${\displaystyle \cos(\theta )=1-2abc/S}$.

Applying this, for example, to the disdyakis triacontahedron (${\displaystyle p=4}$, ${\displaystyle q=6}$ and ${\displaystyle r=10}$, hence ${\displaystyle a=2}$, ${\displaystyle b=3}$ and ${\displaystyle c=\phi +2}$, where ${\displaystyle \phi }$ is the golden ratio) gives ${\displaystyle \cos(\alpha _{4})={\frac {2-\phi }{6(2+\phi )}}={\frac {7-4\phi }{30}}}$ and ${\displaystyle \cos(\theta )={\frac {-10-7\phi }{14+5\phi }}={\frac {-48\phi -155}{241}}}$.

Quadrilateral faces

Of the 13 Catalan solids, 4 have quadrilateral faces. These are of the form Vp.q.p.r, where p, q and r take their values among 3, 4, and 5. The angle ${\displaystyle \alpha _{p}}$can be computed by the following formula:

${\displaystyle \cos(\alpha _{p})={\frac {2\cos ^{2}(\pi /p)-\cos ^{2}(\pi /q)-\cos ^{2}(\pi /r)}{2\cos ^{2}(\pi /p)+2\cos(\pi /q)\cos(\pi /r)}}}$.

From this, ${\displaystyle \alpha _{q}}$, ${\displaystyle \alpha _{r}}$ and the dihedral angle can be easily computed. Alternatively, put ${\displaystyle a=4\cos ^{2}(\pi /p)}$, ${\displaystyle b=4\cos ^{2}(\pi /q)}$, ${\displaystyle c=4\cos ^{2}(\pi /p)+4\cos(\pi /q)\cos(\pi /r)}$. Then ${\displaystyle \alpha _{p}}$ and ${\displaystyle \alpha _{q}}$ can be found by applying the formulas for the triangular case. The angle ${\displaystyle \alpha _{r}}$ can be computed similarly of course. The faces are kites, or, if ${\displaystyle q=r}$, rhombi. Applying this, for example, to the deltoidal icositetrahedron (${\displaystyle p=4}$, ${\displaystyle q=3}$ and ${\displaystyle r=4}$), we get ${\displaystyle \cos(\alpha _{4})={\frac {1}{2}}-{\frac {1}{4}}{\sqrt {2}}}$.

Pentagonal faces

Of the 13 Catalan solids, 2 have pentagonal faces. These are of the form Vp.p.p.p.q, where p=3, and q=4 or 5. The angle ${\displaystyle \alpha _{p}}$can be computed by solving a degree three equation:

${\displaystyle 8\cos ^{2}(\pi /p)\cos ^{3}(\alpha _{p})-8\cos ^{2}(\pi /p)\cos ^{2}(\alpha _{p})+\cos ^{2}(\pi /q)=0}$.

Metric properties

For a Catalan solid ${\displaystyle {\bf {C}}}$ let ${\displaystyle {\bf {A}}}$ be the dual with respect to the midsphere of ${\displaystyle {\bf {C}}}$. Then ${\displaystyle {\bf {A}}}$ is an Archimedean solid with the same midsphere. Denote the length of the edges of ${\displaystyle {\bf {A}}}$ by ${\displaystyle l}$. Let ${\displaystyle r}$ be the inradius of the faces of ${\displaystyle {\bf {C}}}$, ${\displaystyle r_{m}}$ the midradius of ${\displaystyle {\bf {C}}}$ and ${\displaystyle {\bf {A}}}$, ${\displaystyle r_{i}}$ the inradius of ${\displaystyle {\bf {C}}}$, and ${\displaystyle r_{c}}$ the circumradius of ${\displaystyle {\bf {A}}}$. Then these quantities can be expressed in ${\displaystyle l}$ and the dihedral angle ${\displaystyle \theta }$ as follows:

${\displaystyle r^{2}={\frac {l^{2}}{8}}(1-\cos \theta )}$,

${\displaystyle r_{m}^{2}={\frac {l^{2}}{4}}{\frac {1-\cos \theta }{1+\cos \theta }}}$,

${\displaystyle r_{i}^{2}={\frac {l^{2}}{8}}{\frac {(1-\cos \theta )^{2}}{1+\cos \theta }}}$,

${\displaystyle r_{c}^{2}={\frac {l^{2}}{2}}{\frac {1}{1+\cos \theta }}}$.

These quantities are related by ${\displaystyle r_{m}^{2}=r_{i}^{2}+r^{2}}$, ${\displaystyle r_{c}^{2}=r_{m}^{2}+l^{2}/4}$ and ${\displaystyle r_{i}r_{c}=r_{m}^{2}}$.

As an example, let ${\displaystyle {\bf {A}}}$ be a cuboctahedron with edge length ${\displaystyle l=1}$. Then ${\displaystyle {\bf {C}}}$ is a rhombic dodecahedron. Applying the formula for quadrilateral faces with ${\displaystyle p=4}$ and ${\displaystyle q=r=3}$ gives ${\displaystyle \cos \theta =-1/2}$, hence ${\displaystyle r_{i}=3/4}$, ${\displaystyle r_{m}={\frac {1}{2}}{\sqrt {3}}}$, ${\displaystyle r_{c}=1}$, ${\displaystyle r={\frac {1}{4}}{\sqrt {3}}}$.

All vertices of ${\displaystyle {\bf {C}}}$ of type ${\displaystyle p}$ lie on a sphere with radius ${\displaystyle r_{c,p}}$ given by

${\displaystyle r_{c,p}^{2}=r_{i}^{2}+{\frac {2r^{2}}{1-\cos \alpha _{p}}}}$,

and similarly for ${\displaystyle q,r,\ldots }$.

Dually, there is a sphere which touches all faces of ${\displaystyle {\bf {A}}}$ which are regular ${\displaystyle p}$-gons (and similarly for ${\displaystyle q,r,\ldots }$) in their center. The radius ${\displaystyle r_{i,p}}$ of this sphere is given by

${\displaystyle r_{i,p}^{2}=r_{m}^{2}-{\frac {l^{2}}{4}}\cot ^{2}(\pi /p)}$.

These two radii are related by ${\displaystyle r_{i,p}r_{c,p}=r_{m}^{2}}$. Continuing the above example: ${\displaystyle \cos \alpha _{3}=-1/3}$ and ${\displaystyle \cos \alpha _{4}=1/3}$, which gives ${\displaystyle r_{c,3}={\frac {3}{8}}{\sqrt {6}}}$, ${\displaystyle r_{c,4}={\frac {3}{4}}{\sqrt {2}}}$, ${\displaystyle r_{i,3}={\frac {1}{3}}{\sqrt {6}}}$ and ${\displaystyle r_{i,4}={\frac {1}{2}}{\sqrt {2}}}$.

If ${\displaystyle P}$ is a vertex of ${\displaystyle {\bf {C}}}$ of type ${\displaystyle p}$, ${\displaystyle e}$ an edge of ${\displaystyle {\bf {C}}}$ starting at ${\displaystyle P}$, and ${\displaystyle P^{\prime }}$ the point where the edge ${\displaystyle e}$ touches the midsphere of ${\displaystyle {\bf {C}}}$, denote the distance ${\displaystyle PP^{\prime }}$ by ${\displaystyle l_{p}}$. Then the edges of ${\displaystyle {\bf {C}}}$ joining vertices of type ${\displaystyle p}$ and type ${\displaystyle q}$ have length ${\displaystyle l_{p,q}=l_{p}+l_{q}}$. These quantities can be computed by

${\displaystyle l_{p}={\frac {l}{2}}{\frac {\cos(\pi /p)}{\sin(\alpha _{p}/2)}}}$,

and similarly for ${\displaystyle q,r,\ldots }$. Continuing the above example: ${\displaystyle \sin(\alpha _{3}/2)={\frac {1}{3}}{\sqrt {6}}}$, ${\displaystyle \sin(\alpha _{4}/2)={\frac {1}{3}}{\sqrt {3}}}$, ${\displaystyle l_{3}={\frac {1}{8}}{\sqrt {6}}}$, ${\displaystyle l_{4}={\frac {1}{4}}{\sqrt {6}}}$, so the edges of the rhombic dodecahedron have length ${\displaystyle l_{3,4}={\frac {3}{8}}{\sqrt {6}}}$.

The dihedral angles ${\displaystyle \alpha _{p,q}}$between ${\displaystyle p}$-gonal and ${\displaystyle q}$-gonal faces of ${\displaystyle {\bf {A}}}$ satisfy

${\displaystyle \cos \alpha _{p,q}={\frac {l^{2}}{4}}{\frac {\cot(\pi /p)\cot(\pi /q)}{r_{m}^{2}}}-{\frac {r_{i,p}r_{i,q}}{r_{m}^{2}}}={\frac {l_{p}l_{q}-r_{m}^{2}}{r_{c,p}r_{c,q}}}}$.

Finishing the rhombic dodecahedron example, the dihedral angle ${\displaystyle \alpha _{3,4}}$ of the cuboctahedron is given by ${\displaystyle \cos \alpha _{3,4}=-{\frac {1}{3}}{\sqrt {3}}}$.

Application to other solids

All of the formulae of this section apply to the Platonic solids, and bipyramids and trapezohedra with equal dihedral angles as well, because they can be derived from the constant dihedral angle property only. For the pentagonal trapezohedron, for example, with faces V3.3.5.3, we get ${\displaystyle \cos(\alpha _{3})={\frac {1}{4}}-{\frac {1}{4}}{\sqrt {5}}}$, or ${\displaystyle \alpha _{3}=108^{\circ }}$. This is not surprising: it is possible to cut off both apexes in such a way as to obtain a regular dodecahedron.

See also

• List of uniform tilings Shows dual uniform polygonal tilings similar to the Catalan solids
• Conway polyhedron notation A notational construction process
• Archimedean solid
• Johnson solid

References

• Eugène Catalan Mémoire sur la Théorie des Polyèdres. J. l'École Polytechnique (Paris) 41, 1-71, 1865.
• Alan Holden Shapes, Space, and Symmetry. New York: Dover, 1991.
• Wenninger, Magnus (1983), Dual Models, Cambridge University Press, ISBN 978-0-521-54325-5, MR 0730208 (The thirteen semiregular convex polyhedra and their duals)
• Williams, Robert (1979). The Geometrical Foundation of Natural Structure: A Source Book of Design. Dover Publications, Inc. ISBN 0-486-23729-X. (Section 3-9)
• Anthony Pugh (1976). Polyhedra: A visual approach. California: University of California Press Berkeley. ISBN 0-520-03056-7. Chapter 4: Duals of the Archimedean polyhedra, prisma and antiprisms

External links

• Weisstein, Eric W. "Catalan Solids". MathWorld.
• Weisstein, Eric W. "Isohedron". MathWorld.
• Catalan Solids – at Visual Polyhedra
• Archimedean duals – at Virtual Reality Polyhedra
• Interactive Catalan Solid in Java
• Download link for Catalan's original 1865 publication – with beautiful figures, PDF format