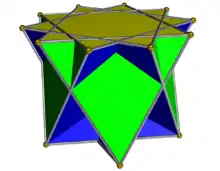
Crossed pentagrammic cupola
In geometry, the crossed pentagrammic cupola is one of the nonconvex Johnson solid isomorphs, being topologically identical to the convex pentagonal cupola. It can be obtained as a slice of the great rhombicosidodecahedron or quasirhombicosidodecahedron. As in all cupolae, the base polygon has twice as many edges and vertices as the top; in this case the base polygon is a decagram.
| Crossed pentagrammic cupola | |
|---|---|
 | |
| Type | Johnson isomorph Cupola |
| Faces | 5 triangles 5 squares 1 pentagram 1 decagram |
| Edges | 25 |
| Vertices | 15 |
| Vertex configuration | 5+5(3.4.10/3) 5(3.4.5/3.4) |
| Schläfli symbol | {5/3} || t{5/3} |
| Symmetry group | C5v, [5], (*55) |
| Rotation group | C5, [5]+, (55) |
| Dual polyhedron | - |
It may be seen as a cupola with a retrograde pentagrammic base, so that the squares and triangles connect across the bases in the opposite way to the pentagrammic cuploid, hence intersecting each other more deeply.
Related polyhedra
| n / d | 4 | 5 | 7 | 8 |
|---|---|---|---|---|
| 3 |  {4/3} |
 {5/3} |
 {7/3} |
 {8/3} |
| 5 | — | — |  {7/5} |
 {8/5} |
The crossed pentagonal cupola may be seen as a part of the uniform polyhedra known as the nonconvex great rhombicosidodecahedron, great dodecicosidodecahedron, and great rhombidodecahedron.
 Crossed pentagrammic cupola |
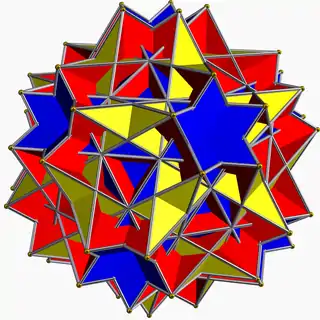 Nonconvex great rhombicosidodecahedron |
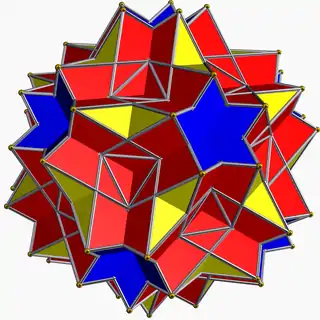 Great dodecicosidodecahedron |
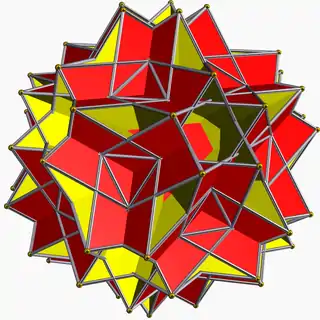 Great rhombidodecahedron |
Various crossed pentagrammic cupolae on the nonconvex great rhombicosidodecahedron may be diminished or gyrated (rotated) to produce a set of 12 polyhedra isomorphic to the Johnson solids J72 to J83. They are the gyrate, metabigyrate, parabigyrate, trigyrate, diminished, metabidiminished, parabidiminished, tridiminished, metagyrate diminished, paragyrate diminished, bigyrate diminished, and gyrate bidiminished quasirhombicosidodecahedra.
Dual polyhedron
The dual of the crossed pentagrammic cupola has 10 triangular and 5 kite faces:

References
- Jim McNeill, Relation of Cupolas to Uniform Polyhedra
- Jim McNeill, Cupola OR Semicupola
- Richard Klitzing, Axial-Symmetrical Edge Facetings of Uniform Polyhedra